Resonant energy link
| Previous chapter ( Torsion field ) | Table of contents | Next chapter ( Illumination ) |
Corresponding Wikipedia article: Wireless power
Introduction
The wireless power transmission is provided by the electromagnetic forces within the transformers and similar systems, which consist of the primary and secondary circuits (coils). One of the main specifications is the efficiency, as a ratio of the secondary power to the primary power.
According to the classical theory, in a case of the great dissipation of the magnetic field, when the circuits are not entirely overlapped in space, and they have the large air gaps, the efficiency is very low. It is mainly determined by a part of the primary magnetic flux, which reaches the secondary circuit, depending on the system design.
The circuit frequency properties (especially resonant) affect the voltage but not the efficiency. The radio transmission over the long distances, which utilizes the resonance, has a negligible efficiency because of the small area of the receiving surface.
Let a transformer consists of two nested solenoids. The secondary coil is inside the primary coil. Because the flux is proportional to a cross-section area of a solenoid and the flux density is almost the same over the cross section, the efficiency does not exceed the square of a ratio of the winding diameters. For example, in case of the diameters difference in two times, the efficiency does not exceed 25%.
Let a transformer consists of two rings, which are spaced apart from each other. The magnetic flux density of a ring of radius \(R\) at a distance \(D\) along the axis of the ring is expressed by the Biot-Savart law so: \[B=\frac{\mu_0}{2}\frac{JR}{R^2+D^2}\tag{1}\] The mutual inductance of two rings at a distance \(D\) from each other, ignoring the inhomogeneity of the magnetic field across the axis, is: \[M=\frac{\Phi}{J}\approx\frac{\pi R^2B}{J}=\frac{\pi\mu_0}{2}\frac{R}{1+(D/R)^2}\tag{2}\] The inductance of the ring of a wire of radius \(r_0\) is: \[L=\mu_0 R\left(\ln\frac{8R}{r_0}-1,75\right)\tag{3}\] The transformation efficiency is mainly determined by the mutual inductance: \[\eta\approx\frac{M}{L}=\left(\frac{\pi}{2\ln\frac{8R}{r_0}-3,5}\right)\left(\frac{1}{1+(D/R)^2}\right)\tag{4}\] Example: Wire of diameter 2 mm (\(r_0\) = 1 mm). Two coaxial rings of radii \(R\) = 5 cm, at a distance \(D\) from each other of 5, 10 and 20 cm. The efficiency values are respectively \(\eta_5\) ≈ 19%, \(\eta_{10}\) ≈ 7%, \(\eta_{20}\) ≈ 2%.
Considering the ring from the given example as an ideal omnidirectional antenna, the efficiency of the radio communication between these rings can be estimated as the ratio of the approximate receptive ring area to the sphere area of a radius \(D\), that is \((2\pi R\cdot 2r_0)⁄(4\pi D^2)=(Rr_0)⁄D^2\). The efficiency values are respectively \(\eta_5\) = 2%, \(\eta_{10}\) ≈ 0,5%, \(\eta_{20}\) ≈ 0,1%. Since these antennas are not focused, the negligibility of the efficiency values does not vary significantly when the antenna directivity is took into account.
Tesla coil
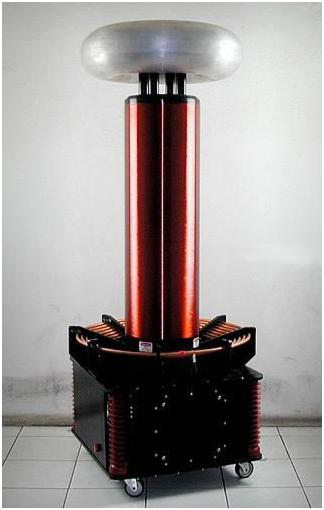
The Tesla coil is a completely irrational design with apparently insignificant efficiency from the point of view of the above examples. This transformer, however, increases the voltage at a ratio sometimes tens times higher than the ratio of turns in its windings, even without a magnetic core. The high efficiency is usually explained only by an electrical resonance in the secondary series LC circuit. Also, they sometimes change the shown classic schematic by a more understandable LC oscillator or an external generator, which is connected to the primary winding. The resonance explains the high voltage, but it does not explain the high efficiency, and it absolutely does not explain such an original design.
The aether theory more comprehensively explains the design and operation of the classic Tesla coil. Thus, the mutual inductance of the windings can be ignored, because mainly the aetheric vortices are transferred between the windings, which is called the radiant energy in Tesla’s terms. The magnetic core is not necessary, because it resists to the growth and motion of these vortices. The vortex is initiated by the annular electromagnetic beams of the spirally shaped primary winding, wherein the interturn electric field vector is almost perpendicular to the magnetic field vector. A slight spiral obliquity directs the vertical vortex component.
The vortex arises and grows with the spark gap breakdown. After the capacitor discharge, the arc in the spark gap disappears and the vortex is not supported. The cycle repeats again after charging the capacitor. The cyclic process is self-oscillating and thus resonant.
The vortices have an electric charge, which induces the charge of an opposite sign in the surrounding conductors, particularly in the grounded secondary winding. The toroidal terminal has the maximum concentration of the charge due to its high capacitance. As a result of the electrostatic attraction, a vortex leaves the primary winding, rises along the secondary winding, gives an energy to it, and then decays.
The secondary winding terminal provides a high voltage, which produces, because of its high frequency, an exotic aura of the electric discharges. The annular vortices reach the toroidal terminal and induce the appropriate eddy currents within it. Also the mobile plasmoids are emitting from this terminal in some cases.
- Plasmoids
The artheric vortices of a Tesla coil improve the carbon nanotubes self-assembly within a strong electric field, and also they transmit power to the LEDs assembled from these tubes [1].
The Tesla coil efficiency theoretically may exceed 100%, because the aetheric vortices consume the ambient aetheric energy, which can be given into the secondary winding.
The efficiency of the resonant and vortical pulse transformers is a ratio of the output aetheric vortical energy, which is consumed by the secondary winding, to the well-known input energy of the vortical magnetic field and the potential electric field, which are producing a vortex. \[\eta=\frac{W_{OUT}}{W_{IN}}\tag{5}\] \[W_{IN}=\frac{1}{2}\int{\left(\frac{B^2}{\mu_0\mu}+\varepsilon_0\varepsilon E^2\right)\mathrm{d}V}\tag{6}\] The energy of an aetheric vortex ("Aetheric vortex", 2) by substituting the density definition ("Mass and inertia", 2) is expressed as: \[W_{OUT}=\int{\rho v^2\mathrm{d}V}=\varepsilon_0\int{\varepsilon B^2v^2\mathrm{d}V}\tag{7}\] Assuming that the beam speed is determined by the medium permeability and permittivity, the vortex energy is: \[W_{OUT}=\int{\frac{B^2}{\mu_0\mu}\mathrm{d}V}\tag{8}\] Also assuming the input and the output magnetic fields the same, the efficiency is estimated as: \[\eta=2\frac{W_{OUT}}{W_{OUT}+\varepsilon_0\int{\varepsilon E^2\mathrm{d}V}}\tag{9}\] According to this simplified model, the resonant transformer efficiency cannot reach and exceed 200%, because they require the electric field energy to create a vortex.
The actual efficiency depends on the actual magnetic and velocity field of the vortices, as well as on the percentage of their hits into the secondary winding, and varies widely. The efficiency dependence on the power is typical.
"Magnetic resonance"
The energy transmission, which is based on the "magnetic resonance", is a flow of the low-power aethric vortices or the torsion waves, which are also called the "non-radiative" magnetic field. The antennas have an annular spiral shape. The vortices, which are produced by the transmitting spiral antenna with the large interturn gaps, are emitted mainly in one direction. These vortices are amplified in the receiving spiral antenna, consuming an aetheric energy and giving it into the load.
This technology cannot be explained from the point of view of classical physics, because a usual electrical resonance, in this case, is not related to the transformation efficiency. However, this technology exists in the prototypes called WREL. The efficiency is 75% at a distance about 1 meter.
References
- ↑ Lindsey R. Bornhoeft, Aida C. Castillo, Preston R. Smalley, Carter Kittrell, Dustin K. James, Bruce E. Brinson, Thomas R. Rybolt, Bruce R. Johnson, Tonya K. Cherukuri, and Paul Cherukuri, Teslaphoresis of Carbon Nanotubes, ACS Nano, 2016, 10 (4), pp 4873–4881
| Previous chapter ( Torsion field ) | Table of contents | Next chapter ( Illumination ) |